Unlocking the Real World: Your Guide to Trigonometry Applications
Ever gazed at a skyscraper or a suspension bridge and wondered about the math behind their construction? Or perhaps you've been captivated by the precise angles and distances involved in sports like basketball or golf? These are just glimpses into the world of applied trigonometry. This isn't just about memorizing SOH CAH TOA; it's about understanding how those ratios unlock solutions to fascinating, real-world problems.
Trigonometry, at its core, deals with the relationships between angles and sides in triangles. But its reach extends far beyond textbook diagrams. When we talk about "trigonometry application problems," we're talking about using these relationships to solve practical scenarios – scenarios you might encounter in fields like architecture, engineering, navigation, physics, and even music!
The roots of trigonometry can be traced back to ancient civilizations like the Egyptians and Babylonians, who used it for surveying land and astronomy. Over centuries, mathematicians developed and refined trigonometric concepts, leading to the sophisticated tools we use today. Why has trigonometry remained so relevant? Because its applications are fundamental to understanding and shaping the world around us.
One of the biggest hurdles people face with trigonometry application problems is bridging the gap between theory and practice. It's one thing to solve for 'x' in an equation; it's another to visualize how that 'x' represents the height of a building or the distance a boat needs to travel. That's where focused practice comes in. By working through a variety of application problems, you train your mind to see the connections between abstract concepts and concrete situations.
Think of a "trigonometry application problems worksheet" not as a dreaded homework assignment, but as a toolbox filled with exciting challenges. Each problem you solve is like adding a new tool to your kit, equipping you to tackle increasingly complex and interesting scenarios.
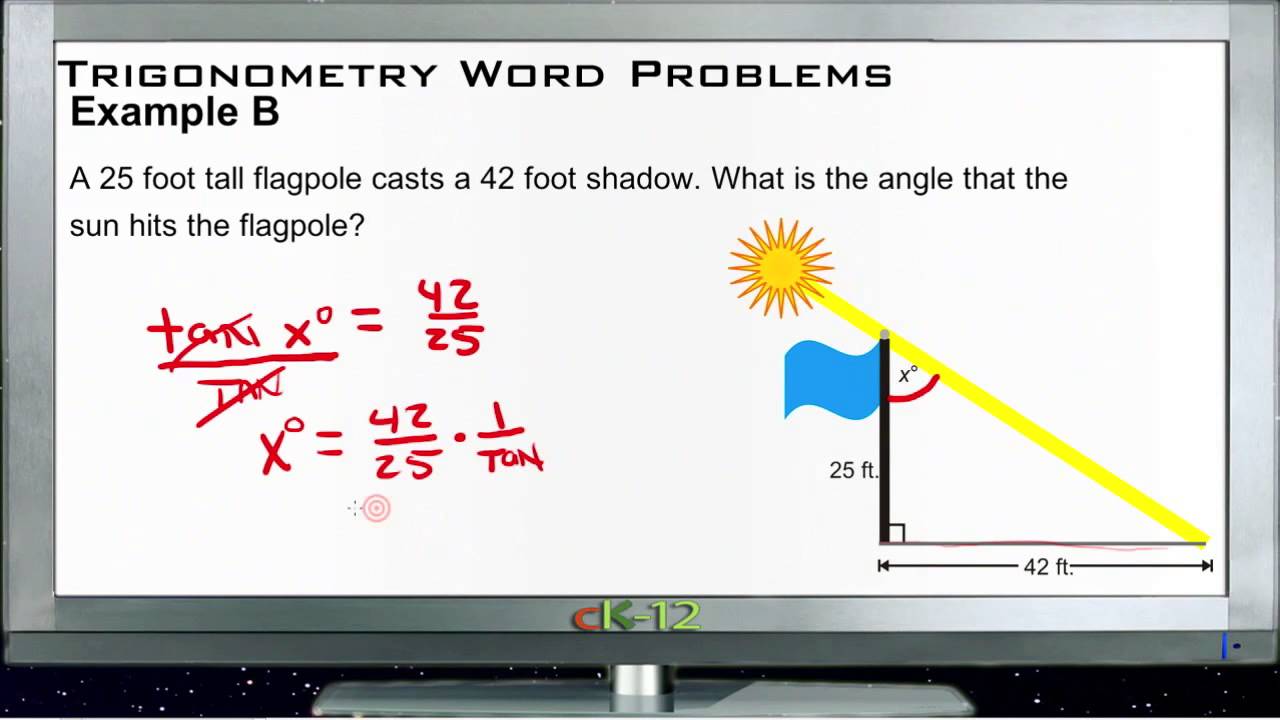
Let's illustrate with a simple example. Imagine you're trying to determine the height of a tree. You can't climb it to measure, but you do have a protractor and a tape measure. By measuring the angle of elevation to the top of the tree from a certain distance and applying the tangent function (SOH CAH TOA, remember?), you can calculate the tree's height. This simple example demonstrates the power of trigonometry in action.
Working through these types of problems offers several key benefits:
1. Enhanced Problem-Solving Skills: Trigonometry applications force you to break down problems into manageable parts, identify relevant information, and apply concepts strategically. This process strengthens your analytical and critical-thinking skills, valuable assets in any field.
2. Deeper Understanding: When you see trigonometry in action, the concepts become more than just formulas on a page. You gain a deeper, more intuitive understanding of the relationships between angles, sides, and distances.
3. Career Relevance: A strong grasp of trigonometry applications is a gateway to a wide range of careers. Whether you're interested in STEM fields, design, or even music, these skills will serve you well.
To make the most of your trigonometry learning journey, consider these tips:
1. Start with the Basics: Ensure you have a solid foundation in the fundamental trigonometric ratios (sine, cosine, tangent) and their inverses.
2. Visualize: Always draw a diagram when solving an application problem. Label the known values and the unknown you're trying to find. This visual aid will help you select the appropriate trigonometric ratio.
3. Practice Regularly: Consistent practice is key. Seek out a variety of problems to challenge yourself and reinforce your understanding.
Advantages and Disadvantages of Using Trigonometry Application Problem Worksheets
| Advantages | Disadvantages |
|---|---|
| Structured learning approach | Potential for repetition and monotony |
| Provides immediate feedback | May not cover all real-world scenarios |
| Allows for self-paced learning | Can emphasize rote memorization over conceptual understanding |
Frequently Asked Questions
1. What are some good resources for finding trigonometry application problems? Textbooks, online educational platforms (Khan Academy, Coursera), and dedicated math websites offer a plethora of practice problems.
2. How do I know which trigonometric ratio to use in a problem? Carefully analyze the given information and the unknown you're solving for. The relationship between those values and the angle will guide your choice of sine, cosine, or tangent.
3. Are there any shortcuts to solving trigonometry application problems? While there are no shortcuts to mastering the concepts, practice helps you develop intuition and recognize patterns, leading to more efficient problem-solving.
4. Can calculators solve these problems for me? Calculators are useful tools, but it's crucial to understand the concepts and the steps involved in solving the problem. Blindly relying on a calculator won't develop your problem-solving skills.
5. Is trigonometry used in everyday life? Absolutely! From simple tasks like hanging a picture frame to more complex applications in fields like navigation and construction, trigonometry is all around us.
6. How important are radians in solving application problems? While many problems can be solved using degrees, understanding radians, a unit of angle measurement, is essential for more advanced applications and higher-level mathematics.
7. What are some common mistakes to avoid when solving these problems? Careless errors in calculations, incorrectly identifying the right trigonometric ratio, and forgetting unit conversions are common pitfalls.
8. How can I get better at solving trigonometry word problems? Start by breaking down the problem into smaller, manageable parts. Identify what is given, what you need to find, and draw a clear diagram. Then, apply your trigonometry knowledge to solve for the unknown.
In conclusion, diving into the world of trigonometry application problems is an exciting endeavor. It transforms theoretical knowledge into practical skills, equipping you to analyze, understand, and solve real-world challenges. From the heights of skyscrapers to the intricacies of sound waves, trigonometry is the key. Embrace the challenge, practice diligently, and unlock a world of possibilities with your newfound trigonometric prowess.
Why is my face rebelling understanding granos en la cara causas
Unlocking creativity the power of cartoon images for kids in png format
Groan worthy goodness exploring the world of dad jokes
